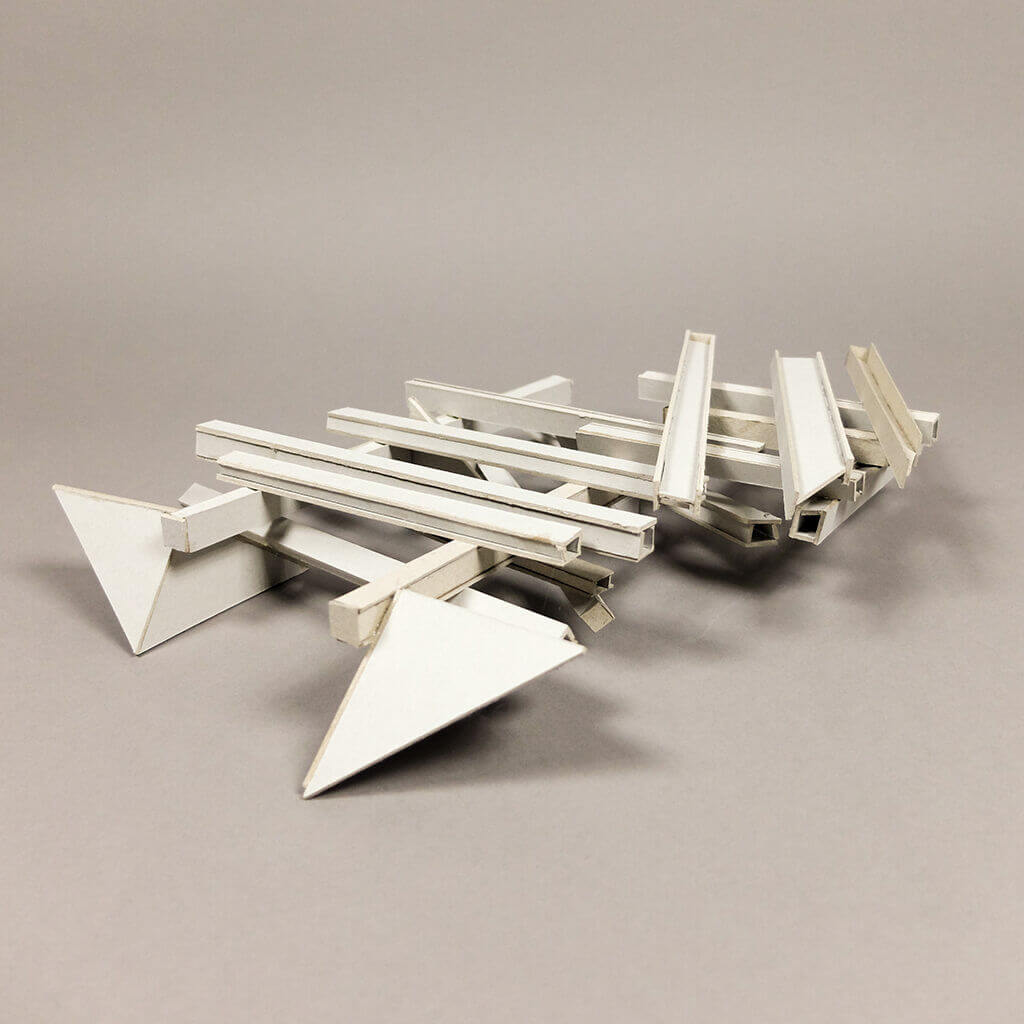
In the Fall 2013 I took on the Chair of Descriptive Geometry at the TU Berlin. Being familiar with the school, my predecessors and their pedagogical ideas, I focused my attention on creating a new dynamic around the question; how to integrate the department into the first year design studios?

Secondly my vision was to create a hybrid form of pedagogy based on traditional hands-on analog skills meshed with the contemporary digital era.

My predecessor’s argumentation was: the study of descriptive geometry is mathematical; its purpose is to produce exact representations of three dimensional (3D) bodies and to solve spatial problems in two dimensions using geometrical means, thereby training the spatial experience necessary to the practice of architecture [2]. While these concepts may be true, I wonder if they aren’t biased and out of touch with today’s digital culture? Lynn suggests “challenging these assumptions by introducing architecture to models of organization that are not inert will not threaten the essence of the discipline, but will advance it, […] an animate approach to architecture will subsume traditional models of statics into a more advanced system of dynamic organization” [3].

These notions combined with my experience working with Prof. Raimund Abraham of Cooper Union in New York City lead me to question the pedagogical means of teaching spatial cognition to freshman students of architecture.

The outcome to some of these questions is reported in this paper. It focuses its attention on teaching students how to decode (to decipher) and to encode (to convey symbolically) architectures. The paper explores four didactic methods, lectures, autodidactic study, exercises conveying digital and analog tools, and using these tools sets in the first year design studio. The goals focus on describing spatial relationships while aiding pupils to discover and refine their own architectural language.

My first move upon taking over the TU Berlin’s Descriptive Geometry (DG) department was to rename it. I purposefully chose the word “architectonics”, meaning: “the scientific study of architecture” [4] and since the word “tectonic” implies structure, it seemed appropriate as I am researching the semantics of architecture.

The ultimate goal is: to hand down a set of tools to students and teach them to see, or in other words, how to read architecture, to then translate it into a new architecture; hence the ideas of decoding and encoding.

Referring to the seminal texts from our architectural heritage, we discover architects busy recording and decoding their predecessors: Vitruvius studied the Greeks, Palladio studied Vitruvius, Viollet-le-Duc studied the Middle Ages, etc. Finally, Le Corbusier hit a wall and found himself at a crossroads between the Greeks and his contemporary engineering culture of machines. Rem Koolhaas was later forced to explore the verticality of architecture, its compactness and layering. The point is we are obsessed with learning from past and confronted by the present in order to develop something new. These are some examples of decoding architecture.

Encoding architecture is just as exciting. For instance: Palladio took his Vitruvian research and encoded it into what we know as Palladian architecture, Peter Eisenman took Palladio and made House X; Henri Labrouste’s Bibliothèque Sainte-Geneviève appears to be greatly influenced by his contemporary, Viollet-le-Duc’s anthology of medieval and gothic architecture (Dictionnaire raisonné de l’architecture française du XIème au XVIème).

So when and where have architects learned how to decode and encode architecture?

While there exists analysis courses taking apart buildings to understand their programmatic structures and others search for proportional systems, it appears few reduce architecture to its spatial study without delving into diagrams or dry mathematical theorems.

While evaluating the work of Preston Scott Cohen, Moneo compellingly reinforces notions that “geometry can be a tool to deconstruct memory into another set of ideas and that projective geometry can transcend and transform the burdensome questions of type” [5]. This suggests descriptive or projective geometry could be used to investigate the semantic qualities of architecture.

I would argue the idea of deconstructing memory is a key to learning how to decode architecture.

Abraham refers to Heidegger’s “Ort” (a site, a place, a point) so infinitely small where all forces and vectors are concentrated and liberated, released as energy, transformed into matter into built form. Abraham proposes “the transformation becomes the history of the site as place, while the cognition of forces and vectors becomes the memory of events […]. The manifestation of the memory of spatial events from history of the site is idealized through the abstraction of the language of architecture.” Abraham’s conclusion is “architecture becomes the idealized language of historical associations whereby ideal ought to be defined as a form of radical clarity.” [6]

Learning to encode architecture is a long process; however it can be accelerated by remaining in an abstract procedural environment jumping in and out of imaginary space, physical space and virtual space while confronting it with practical moments. The three following didactic methods: lectures, exercises and autodidactic research are used as foundations which are built upon in the design studio.

Lectures
To communicate these ideas to students, I developed a lecture series reinforcing the culture of drawing, the visual communication of space and architecture as demonstrated in the writings and drawings of the Masters. Unlike my predecessors that spent their time drawing the mathematical rules of projective geometry on the chalkboard, I chose to visually and dynamically explain the origins, philosophical implications, the tools and the architectures.

The lecture series meandered through decoding and encoding, light and shadow, the history of geometry, indexical and combinatorial techniques, the status quo, perspective from many perspectives, to name a few. The lectures are online at our website www.fgbarlieb.de and have the particularity of pointing the users to highly detailed digital scans of original facsimile found in libraries around the world. Being a collector of original manuscripts, I believe it is important students gain access to digital facsimile of seminal works to grasp the dedication necessary to producing and revealing architectural space.

Exercises
The following exercises are a case study put into practice during the Fall semester of 2013-2014 at the TU Berlin Institute for Architecture (IfA) to train students in the discipline of architecture and of applying the training back onto their first year design studio.

1. Dot Zero: is the first of a series of exercises developed to address the practice of decoding and encoding space. A Cartesian coordinate composed of the X,Y & Z axes (no larger than 50x50x50 cm, Fig. 1) is designed to be portable and built of resilient materials by the student. The resulting precision tool is used to measure and record an architectonic space.

Fig. 1 – Dot Zero. T. Hemig

2. Recording Space: A Cartesian coordinate composed of the X,Y & Z axes is used to measure and record an architectonic space. The student carefully place the Dot Zero tool in space and record a series of six black and white photographs along each of the individual axes (+X, -X, +Y, -Y, +Z, -Z). Black and white photography is chosen to emphasize the spatial qualities by concentrating on light and shadow

3. Tracing (cropping, cartography, codex of points, lines and planes): The six photographs are printed on DIN A3 format sheets of paper. Three sheets of double sided Mylar 28cm2 (Fig. 2) are used to crop the images and focus on generating new compositions. The students are required to decode their photographs by tracing key geometries and to develop an architectural codex of lines (solid, dashed, dotted, dash-dot, etc). Each Mylar sheet is perceived as a “window pane” so that one side of the pane is the positive direction of an axis while the reverse side is the negative direction of the corresponding axis.

This leads to a disciplined study with pencil, paper, compass and parallel ruler drafting tools, dashed lines are drawn as if sewn by a machine and line weights and erasing techniques are explored to highlight the tectonic qualities of drawing.

Fig. 2 – Pencil on Mylar. D. Ortner

4. Analog to Digital (scan, points, vectors, lines, splines and planes): In a subsequent exercise the drawings are digitally scanned and traced using vector based drawing tools such as Adobe Illustrator thus focusing on pure vector based software instead of creating confusion by introducing more complex CAD software. Essentially we are focusing on understand what the difference is between a vector and a bitmap while promoting the idea that hand drawings can be rapidly scanned into the computer and traced accordingly.

Fig. 3 – Parametric Model. B. Rusch

5. Project and Control (export, import, algorithm, encoding space): The Illustrator drawings are then imported into Rhinoceros CAD software using the DWG CAD file format and reassembled in virtual space using Grasshopper visual scripting components. This exercise is the first time students interact with a powerful parametric design tool. Because the design space is simple (a point, a vector, a picture plane and a projection plane) and contains a vast amount of data, the students rapidly realize its power and logic.

This visual form of scripting is essential to architects as they are not trained as mathematicians in abstract scripting languages; instead they demand and require visual spatial cues for composition. The iterative nature of the environment enables students to rapidly experiment with variations of their studies and to visualize them in 3D for the first time (Fig. 3). It is also the chance to address the concept of the algorithmic in architecture. What’s more is the students are controlling the tool; the tool is not controlling the students or their work! The arguments that digital experimentation can lead to new architectures is a wonderful concept yet I believe they are more relevant to Master students pursuing research versus Bachelor students learning the foundations of the discipline of architecture. This may shift with the advent of new media or new spatial cognition theories.

Fig. 4 – Paper Model. S. Schoelhammer

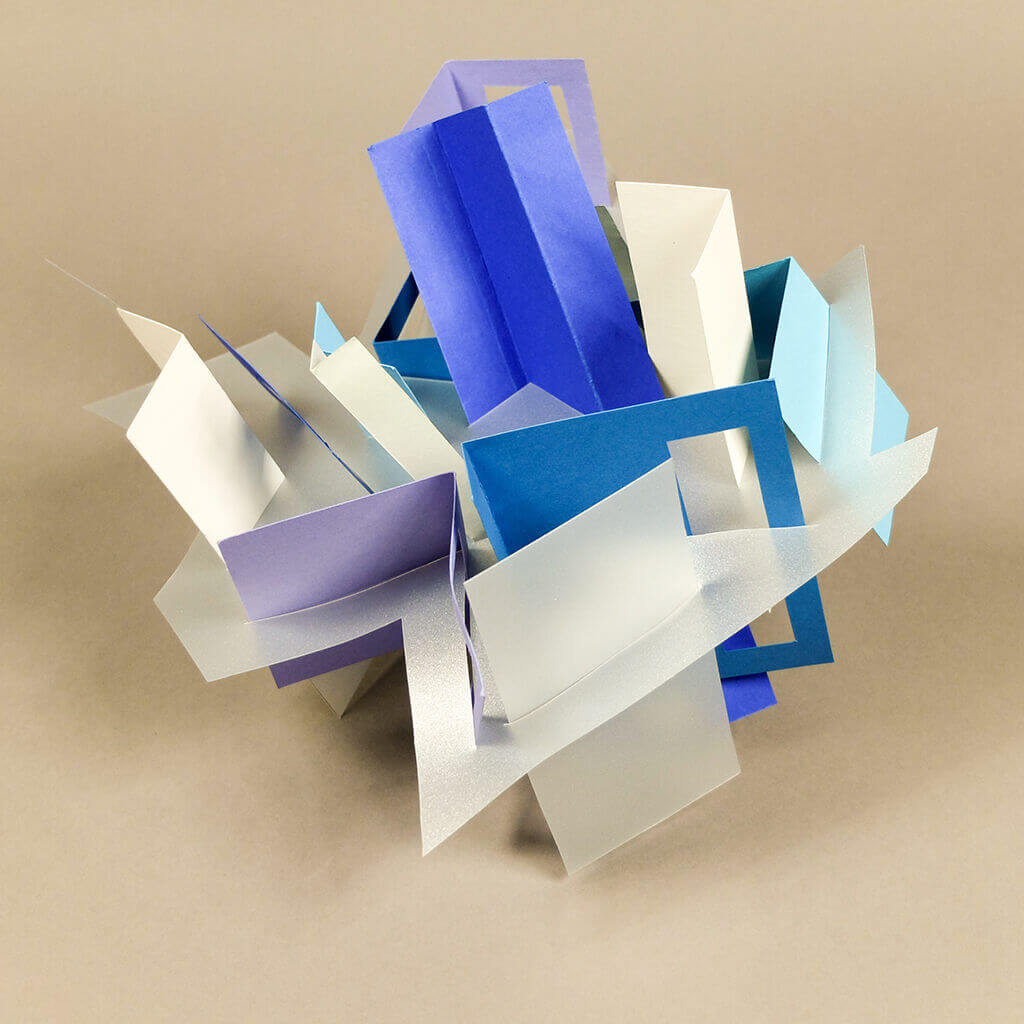
6. Modeling: The decoded line drawing window panes are used to encode and build three dimensional paper models by defining what the lines could mean i.e.: cutting, scoring, creasing, folding, and bending (Fig. 4). The models are purposefully made of paper to address the concepts of flat stock fabrication which they revisit in their second semester while building a 1:1 prototype of one of their models.

7. Light & Shadow: The models are illuminated in a photo studio environment to demonstrate composition and exposure of a photo camera picture plane. The new images are used to gain practical experience with shadow casting and are reversed engineered by students to learn about light projection geometry and shadow drawing techniques.

Autodidactic research
Descriptive geometry seems like it’s the perfect autodidactic course. Since I rebuilt the entire department to serve the purpose of decoding and encoding architecture; I figured the best way the students would learn descriptive geometry terms such as: projection, platonic solids, conical sections, splines, freeform geometry, axonometry would be on their own time, studying in the library so we could spend our studio time learning how to draw, translate and transform architecture. This turned out to be a success and we’ve taken the best redacted works on the various topics and edited them into a book for the students by the students.

The aforementioned forms of study relative to the discipline of architecture are required foundations for first year architecture students. The design studio is the space to put these notions into practice. Students begin understanding that what they draw is architecture, and not a representation of architecture!

In essence we are teaching the students to craft space using the architectural vocabularies, grammars and syntax along with the codex of points, lines, planes, curves and surfaces. The meshing of digital and analog tools along with procedural modes of transformation leads to and develops abstract and logical forms of spatial cognition. My goal is to pursue this hybrid form of creation by promoting the long tradition of hand crafted architecture while experimenting with new digital media and its possibilities in making new architecture; even as architecture appears to be leaning towards self-generated systems, its creation will never be separate from its media or its generators.

[1] Barlieb, Chr., Pfeiffer, S. Rule Based Design Workshop at the TU Berlin,
Design Modelling Symposium, UdK, 2009.
[2] Bonnani, A. Darstellende Geometrie I.
Ein- und Zweitafelverfahren für Architekten, TU Berlin, 1985.
[3] Lynn, G. Animate Form, Princeton Architectural Press, 1999.
[4] Oxford Dictionaries, Oxford University Press, Retrieved April 16, 2014, from www.oxforddictionaries.com/definition/english/architectonic
[5] Moneo, R. Geometry and the Mediation of Architectural Conflicts: Comments on the Work of Scott Cohen, Contested Symmetries the Architecture Writings of Preston Scott Cohen, Princeton Architectural Press, 2001.
[6] Abraham, R. In Anticipation of Architecture / Fragmentary Notes, [Un]Built ed. Groihofer, B. Springer-Verlag/Wien 1996, 2011.

I wish to thank my teaching colleagues of FG BARLIEB // Architectonics at the TU Berlin IfA: C.Høgsbro, K.Müller, M.Seitz, our tutors K.Blömers, N.Schüller, V.Lang and R.Weisgärber and secretary K.Krampe for their unconditional support and constructive criticism. I am indebted to Prof. Donatella Fioretti for kindly lending me her office and to Prof. Ralf Pasel for trusting and inviting me to teach at the TU Berlin’s IfA.